Sudoku Variants
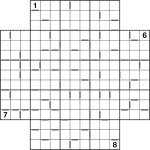
Two-away Jigsaw 8×8
Jul 20th
Continuing with Two-away puzzles, I thought today I’d post a somewhat smaller challenge – an 8×8 Jigsaw Sudoku version.
The rules are pretty much what you’d imagine: place 1 to 8 into each row, column and bold-lined jigsaw piece. Numbers with a grey bar between them must have a difference of 2 (e.g. 1&3) and numbers without a grey bar between must have a difference that’s not 2. (This is important to ensure a unique solution!)
Good luck! ![]()
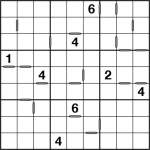
Two-away Samurai Jigsaw
Jul 17th
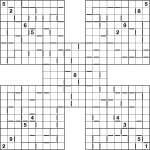
Two-away Samurai Jigsaw puzzle
Here’s a puzzle for the weekend – it’s a 5-grid samurai sudoku where in the corner grids a couple of the 3×3 regions have had their outlines tweaked to turn them into jigsaw sudoku puzzles, with full 8-way symmetry. There are very few givens, which means you’ll need to take full advantage of the two-away markers that are also in the grid. The grey rectangles indicate all neighbouring squares where the difference is 2 (e.g. 1&3 or 6&8) – even without precise values they can also be useful for quickly indicating where a chain of squares are all odd or all even.
Good luck! ![]()
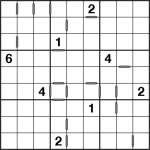
Two-away Samurai Star
Jul 16th
I made this puzzle yesterday but then went and forgot to post it (oops) so I’m making up for that now! It’s a Samurai Star with two-away markers, just as per the previous two puzzles I posted.
Place 1 to 9 into each of the rows, columns and 3×3 boxes of the 5 underlying Sudoku grids (including the one in the centre), whilst obeying the two-away grey bars. Squares with a grey bar between have a difference of 2 (e.g. 1&3 or 2&4), and those without a grey bar have a difference which is not 2.
There are only 4 givens to get you going, so good luck! ![]()
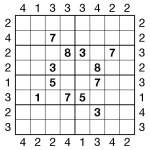
Easy Two-away Sudoku
Jul 9th
I thought that yesterday’s puzzle wasn’t the best example of a new variant, so here’s an easier Two-away Sudoku puzzle. It has a few more connected squares which means the odd/even nature of the puzzle comes through a bit more clearly – if you’re familiar with consecutive sudoku then it probably won’t take you very long to solve.
The rules are as yesterday: place 1 to 9 into each row, column and 3×3 box, and then wherever a grey bar separates two squares the difference must be 2 – they must be “two-away” – and conversely where there is no grey bar then the difference is not 2.
Good luck! ![]()
Two-away Sudoku
Jul 8th
I just noticed that Monday marked my 50th post to garethmoore.co.uk, so to celebrate I thought I’d try a (slightly) new variation today: Two-away Sudoku. As its appearance might suggest, it is a close relative of Consecutive Sudoku. In fact it’s pretty much the same, except that the shaded grey bars indicate that the difference between neighbouring squares is 2 (not 1, as in Consecutive Sudoku). As in Consecutive Sudoku, all grey bars are drawn – so if one is absent then the difference is not 2.
For example, if you see a grey bar next to a square with ‘1′ in it then the neighbouring square must be ‘3′. However if you have a square with ‘1′ in and there is no grey bar between it and a neighbouring square then that square cannot contain ‘3′. It’s pretty simple really.
So – just place 1 to 9 into each row, column and 3×3 box whilst obeying the grey two-away markers!
Good luck! ![]()
Wrap-around Consecutive Samurai Star
Jul 6th
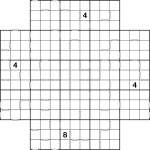
Wrap-around Consecutive Samurai Star puzzle
I seem to be posting more infrequently than I intend, so I thought I’d compensate with a puzzle that would take somewhat longer to complete! Here, then, is a wrap-around consecutive samurai star. All squares with consecutive values (a difference of 1) are marked with white bars, including those at opposite ends of rows and columns – wherever there isn’t a bar, the values are not consecutive.
The Sudoku logic takes a few twists here and there – in fact at one point near the end you will need to spot a particularly nasty hidden set in one region.
To solve the puzzle place 1 to 9 into each row, column and 3×3 box of each of the underlying 5 Sudoku grids (including the one in the centre). You’re only given 4 givens to get going, but with the consecutive information that’s all you need to find a unique solution.
Good luck! ![]()
Skyscraper Sudoku 8×8
Jun 19th
Continuing my series of 8×8 Skyscrapers and variants, here’s an 8×8 Sudoku with Skyscraper clues around the edge.
The rules are simple:
- Place 1 to 8 in each row, column and 4×2 box
- Obey the Skyscraper constraints: each number outside the grid reveals the number of ‘visible’ digits looking along that row/column, where higher digits obscure lower ones
Good luck!
Wrap-around Consecutive 3-grid 6×6 Samurai Skyscraper
May 18th
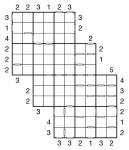
Wrap-around Consecutive 3-grid 6×6 Samurai Skyscraper puzzle
Another mouthful of a puzzle name, but in essence simply a development of the previous puzzle I posted. This time we still have the wrap-around consecutive-ness, but I’ve extended it to a samurai puzzle and added in skyscraper clues. To keep it reasonable, I’ve reduced the underylying Sudoku size to 6×6, however!
What’s particularly fun about the wrap-around markers is that they warp from one side of the puzzle to the same row/column on the opposite side – for the centre two columns this means that they constrain the values of two numbers 10 squares apart.
So the full rules are:
- Place 1 to 6 into each row, column and 2×3 bold-lined box of each of the three underlying 6×6 grids
- White bars show that adjacent cells are consecutive – i.e. 1&2, 2&3, 3&4, 4&5 or 5&6; those squares without a white bar between are non-consecutive
- White bars are shown where appropriate even on the edges of the grid – they indicate how the cell relates to the square at the far end of this row/column of numbers. Remember that the lack of such a white bar means that these wrap-around squares are non-consecutive.
- Skyscraper clues reveal how many numbers can be ’seen’ from that clue number counting in along the adjacent row/column, where higher numbers obscure all lower numbers (see previous puzzles for more detailed instructions)
Just to clarify, if adjacent numbers are equal (which is possible if they’re at far sides of the grid from one another) then these count as non-consecutive.
Good luck! ![]()
Consecutive Wrap-Around Sudoku
May 16th
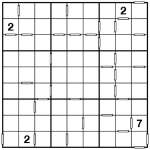
Consecutive Wrap-around Fiendish Sudoku puzzle
Here’s a slight twist on Consecutive Sudoku – I’ve included ‘wrap-around’ consecutive indication, where rows that start and end in consecutive digits have a white bar at the start and end of the row to show this, and similarly for columns. If they’re non-consecutive then there is no white bar. Similarly between all other squares: a white bar indiciates that two squares are consecutive, and no white bar means that they are non-consecutive.
‘Consecutive’ means that the difference between the numbers is ‘1′, i.e. they are 1&2, 2&3, 3&4, 4&5, 5&6, 6&7, 7&8 or 8&9. Other than this it’s a regular Sudoku – place 1 to 9 into each row, column and bold-lined 3×3 box.
This is also a difficult Sudoku, so if you can solve it under 20 minutes that would definitely be good going. Don’t forget about the non-consecutiveness – this is very important to reach the solution, and don’t forget about the wrap-around!
Good luck!
Double Toroidal Killer Sudoku Pro 8×8
May 13th

Double Toroidal Killer Sudoku Pro 8×8 puzzle
This puzzle certainly won’t be to everyone’s tastes, but it’s a double toroidal killer sudoku pro. Why double? Because there are toroidal jigsaw-shaped Sudoku regions, and there are toroidal jigsaw-shaped Killer Sudoku Pro cages. In other words, some of them wrap around the edges of the puzzle. It’s also an 8×8 puzzle, just to keep the Killer Sudoku (Pro!) logic fresh.
The rules are as you might expect:
- Place 1 to 8 into each row, column and bold-lined shape – some of these shapes are toroidal, so wrap from the end of one row/column to the opposite end of the same row/column
- Place numbers into the dashed-line Killer Sudoku Pro cages so that when the given operation is applied the result is the stated number. For ‘-’ operations start with the highest number.
- Numbers can’t repeat in dashed-line cages, and some cages (just one in this puzzle actually) are toroidal, so wrap around the rows/columns as described above
And that’s it! ![]() Good luck!
Good luck!
Hints: As always with this sort of puzzle, if you shade in the 8 bold-lined regions first with different colours you’ll find it much easier to keep track of what you’re doing. Also, all of the regions (both killer cages and bold-lined regions) have rotational symmetry order 4 – in other words, the same pattern repeats 4 times; this might help!


Recent Comments