Posts tagged Killer CalcuDoku
Samurai Killer Calcudoku
Jan 28th

Samurai Killer Calcudoku puzzle
I haven’t posted much here recently because I’ve been spending my time on Sudoku Xtra, so here’s a large puzzle to fill the void a bit.
This is a five-grid Samurai Killer Calcudoku:
- Place 1 to 9 into each row, column and 3×3 box of the five underlying 9×9 Sudoku grids
- Place numbers into the Calcudoku dashed-line cages to fulfil the results at the top-left of each cage. The given operator when applied between all of the numbers must give the stated result, e.g. the solution to “5+” could be “2+1+2″. For subtraction and division start with the largest number, so for example “3-” could be “6-3″.
- Numbers can be repeated in Calcudoku cages, subject to the row, column and 3×3 box constraints.
Unlike my other puzzles I haven’t used any symmetry in this one, but I’m not sure it’s really that obvious on a puzzle like this one. It’s not especially hard, but with so many places to potentially go it might take you a little while.
Good luck! ![]()
Samurai 5-grid Killer CalcuDoku puzzle
May 4th

Samurai 5-grid Killer Calcudoku puzzle
Here’s an interesting puzzle. It’s a 5-grid Samurai Killer CalcuDoku, which means that it has the 3×3 boxes from Killer Sudoku but otherwise works like a CalcuDoku puzzle, albeit a 5-grid Samurai one! All of the operations in this puzzle are addition, so aren’t shown.
Can you place 1 to 9 into each row, column and 3×3 box of each of the underlying 9×9 Sudoku grids, whilst also placing numbers so that each inner cage adds up to the total given at its top-left corner? Numbers can be repeated within these cages (pretty obviously, given how large some of them are!).
There are quite a lot of single digit cells, suggesting (truthfully) that this isn’t actually a very difficult puzzle – but it’s a good proof of concept, I think. You can create really huge cages if you want, without making the puzzle difficult (of course, the easiest way to solve these is to essentially ignore the cage completely, or at least until it is nearly finished). This particular puzzle does not require you to do any complex maths at all.
Good luck!
As Easy as 11, 22, 33… a Killer CalcuDoku
May 3rd

As easy as 11, 22, 33 Killer CalcuDoku
Here’s a relaxing puzzle for a Sunday… or maybe not! Can you complete this Killer CalcuDoku puzzle made up of 1s, 2s and 3s?
Place 11, 12, 13, 21, 22, 23, 31, 32 or 33 into each square so that the result of applying the specified operation to each cage is the given number. (Start with the highest number in the cage for subtraction and division cages). Also, can you obey the standard Sudoku constraints: place each of the 9 different numbers once per row, column and bold-lined 3×3 box? You can repeat numbers within a cage, however, if you wish (which is why it’s a Killer CalcuDoku, not a Killer Sudoku Pro, in my terminology! It’s also why it has solid cages, rather than dashed-line cages).
The logic isn’t too tricky, but for speed you might find a calculator helps you make a few of the logically easy deductions… ![]()
Good luck!
Killer CalcuDoku +/-
May 2nd

Killer CalcuDoku +/- 6×6 puzzle
There’s a lot of very interesting puzzle space to explore between the extremes of Killer Sudoku and KenKen (a trademark of Nextoy LLC, so I will always refer to this as CalcuDoku from now on, unless anyone suggests a better name!).
I’m going to define two in-between puzzles, giving a continuum like this:
- Killer Sudoku
- Killer Sudoku Pro – Killer Sudoku with extra operations (+, -, x, /)
- Killer CalcuDoku – Killer Sudoku Pro with repeated digits in cages, like CalcuDoku
- CalcuDoku – Killer CalcuDoku puzzles without box constraints (e.g. no 3×3 boxes)
To avoid confusion I’m going to draw Killer Sudoku and CalcuDoku the way they always are – with dashed-line cages in the first case and bold lines between squares for the latter (replacing the traditional Sudoku bold lines). Then to distinguish the others, Killer Sudoku Pro will appear exactly like Killer Sudoku except that there will be additional operators within the grid (for operator-less ones I’ll include a question mark “?” or similar after each clue). Killer CalcuDoku, meanwhile, will appear exactly like today’s puzzle – with solid cages within the main puzzle.
Now just to spice things up further, I’m going to mess around with how the puzzles work. Remember that the key difference between Sudoku and Killer Sudoku is that the digits now actually have value as well – so by fiddling with those values we can create an infinite range of new puzzles that solve in quite different ways.
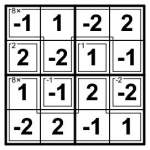
Example Killer CalcuDoku +/- solution
Today is a good example: here’s a 6×6 +/- Killer CalcuDoku. The aim is to place -3, -2, -1, 1, 2 and 3 into each row, column and 2×3 bold-lined box, and to place numbers so that the inner cages compute to the value given when applying the stated operation to the set of numbers in that cage. Subtraction and division are again defined as starting with the highest number in that cage (so remember that 2>-3!) and then applying all the other numbers in any order – so for example the solution to a “4-” cage could be “1 and -3″. Confused? See, I said it would mix things up! (1 – -3 = 4)
I’ve included an example (trivial) 4×4 Killer CalcuDoku +/- solution so you can be sure you understand how it works. But you might not need it – it’s actually a very gentle puzzle I’ve attached, as you’ll probably soon find out… (well, once you get your head around the negative numbers!)
Good luck!

Recent Comments