Posts tagged Sudoku
Samurai 5-grid Killer CalcuDoku puzzle
May 4th

Samurai 5-grid Killer Calcudoku puzzle
Here’s an interesting puzzle. It’s a 5-grid Samurai Killer CalcuDoku, which means that it has the 3×3 boxes from Killer Sudoku but otherwise works like a CalcuDoku puzzle, albeit a 5-grid Samurai one! All of the operations in this puzzle are addition, so aren’t shown.
Can you place 1 to 9 into each row, column and 3×3 box of each of the underlying 9×9 Sudoku grids, whilst also placing numbers so that each inner cage adds up to the total given at its top-left corner? Numbers can be repeated within these cages (pretty obviously, given how large some of them are!).
There are quite a lot of single digit cells, suggesting (truthfully) that this isn’t actually a very difficult puzzle – but it’s a good proof of concept, I think. You can create really huge cages if you want, without making the puzzle difficult (of course, the easiest way to solve these is to essentially ignore the cage completely, or at least until it is nearly finished). This particular puzzle does not require you to do any complex maths at all.
Good luck!
As Easy as 11, 22, 33… a Killer CalcuDoku
May 3rd

As easy as 11, 22, 33 Killer CalcuDoku
Here’s a relaxing puzzle for a Sunday… or maybe not! Can you complete this Killer CalcuDoku puzzle made up of 1s, 2s and 3s?
Place 11, 12, 13, 21, 22, 23, 31, 32 or 33 into each square so that the result of applying the specified operation to each cage is the given number. (Start with the highest number in the cage for subtraction and division cages). Also, can you obey the standard Sudoku constraints: place each of the 9 different numbers once per row, column and bold-lined 3×3 box? You can repeat numbers within a cage, however, if you wish (which is why it’s a Killer CalcuDoku, not a Killer Sudoku Pro, in my terminology! It’s also why it has solid cages, rather than dashed-line cages).
The logic isn’t too tricky, but for speed you might find a calculator helps you make a few of the logically easy deductions… ![]()
Good luck!
Killer CalcuDoku +/-
May 2nd

Killer CalcuDoku +/- 6×6 puzzle
There’s a lot of very interesting puzzle space to explore between the extremes of Killer Sudoku and KenKen (a trademark of Nextoy LLC, so I will always refer to this as CalcuDoku from now on, unless anyone suggests a better name!).
I’m going to define two in-between puzzles, giving a continuum like this:
- Killer Sudoku
- Killer Sudoku Pro – Killer Sudoku with extra operations (+, -, x, /)
- Killer CalcuDoku – Killer Sudoku Pro with repeated digits in cages, like CalcuDoku
- CalcuDoku – Killer CalcuDoku puzzles without box constraints (e.g. no 3×3 boxes)
To avoid confusion I’m going to draw Killer Sudoku and CalcuDoku the way they always are – with dashed-line cages in the first case and bold lines between squares for the latter (replacing the traditional Sudoku bold lines). Then to distinguish the others, Killer Sudoku Pro will appear exactly like Killer Sudoku except that there will be additional operators within the grid (for operator-less ones I’ll include a question mark “?” or similar after each clue). Killer CalcuDoku, meanwhile, will appear exactly like today’s puzzle – with solid cages within the main puzzle.
Now just to spice things up further, I’m going to mess around with how the puzzles work. Remember that the key difference between Sudoku and Killer Sudoku is that the digits now actually have value as well – so by fiddling with those values we can create an infinite range of new puzzles that solve in quite different ways.
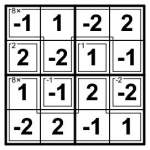
Example Killer CalcuDoku +/- solution
Today is a good example: here’s a 6×6 +/- Killer CalcuDoku. The aim is to place -3, -2, -1, 1, 2 and 3 into each row, column and 2×3 bold-lined box, and to place numbers so that the inner cages compute to the value given when applying the stated operation to the set of numbers in that cage. Subtraction and division are again defined as starting with the highest number in that cage (so remember that 2>-3!) and then applying all the other numbers in any order – so for example the solution to a “4-” cage could be “1 and -3″. Confused? See, I said it would mix things up! (1 – -3 = 4)
I’ve included an example (trivial) 4×4 Killer CalcuDoku +/- solution so you can be sure you understand how it works. But you might not need it – it’s actually a very gentle puzzle I’ve attached, as you’ll probably soon find out… (well, once you get your head around the negative numbers!)
Good luck!
Killer Sudoku Pro / All Signs
May 1st

Killer Sudoku All Signs puzzle
Here’s a Killer Sudoku with “all the signs”, or Killer Sudoku Pro if you prefer. If you’ve got any ideas for a better name let me know! ![]()
As in a regular Killer puzzle, you can’t repeat a digit within the solution to any cage. Unlike in a regular Killer, all non-single cages specify the operation that is applied to produce the result given. If the operation is + or x then just add or multiply all the digits to give that total. For subtraction and division you must start with the largest number in the region and subtract or divide the other numbers from that to give the stated result (this is what you’d do intuitively, I think, but since these operations aren’t commutative it’s necessary to state this!) Other than that, regular Sudoku rules apply: place 1 to 9 into each row, column and bold-lined 3×3 box.
The logic is once again simple, as a first example of this puzzle type, but I think the wider range of operations brings a freshness to the puzzle. Let me know if you agree (or disagree!).
Good luck!
Killer Sudoku Multiplication
Apr 30th

Killer Sudoku Multiplication 9×9 puzzle
Killer Sudoku Multiplication 6×6 puzzle
I thought it would be interesting to see what a Killer Sudoku puzzle would look like if every operation in it was multiplication, so I decided to try it out.
The puzzles attached aren’t labelled with ‘x’ signs, but it should be fairly obvious from the totals that addition is not enough! Single-cell regions are simply equal to the stated value, but in all others you must multiply all of the cell values together to give the total at the top-left. Note that this follows Killer Sudoku rules, so a number cannot be repeated within a region.
These are all definitely rated ‘gentle’ – the logic required is simple, even though the multiplication might appear intimidating! In actual fact you don’t need to calculate most of the big values – try the 6×6 puzzle first to see why this is.
Good luck!
PS Subtraction and division are less interesting, unless you allow negative or fractional numbers! I’ll probably post examples of both the next couple of days anyway however!
Samurai Star Killer Sudoku
Apr 29th
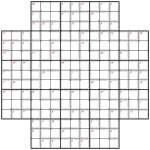
Samurai Star Killer (gentle) puzzle
“Star Killer” sounds like something out of science fiction, but it’s now definitely reality with this 5-grid Killer Sudoku puzzle. The actual Killer part uses the most basic logic imaginable, and there are a lot of ’singleton’ regions which I’ve never used in a Killer puzzle before. The reason for this is that I wanted to start at a gentle level – as a result this mostly solves like a regular Samurai Star (a.k.a. Flower Samurai) puzzle, with the Killer regions used occasionally to either get you going or help you out with a quick number along the way. It shouldn’t take you much over 20 or 30 minutes if you’ve solved this shape of Samurai before, and know what a Killer Sudoku is!
The rules are pretty simple: place 1 to 9 into each row, column and bold-lined 3×3 shape of each of the 5 underlying 9×9 grids (there’s one in the centre too), whilst also placing numbers so that the total in each dashed-line cage is equal to that given in the top-left corner. You may not repeat a number within a dashed-line cage.
The puzzle has rotation symmetry order 4, so the cages are in a pleasing pattern I hope – I particularly like the hole in the square in the centre! I think by and large that if you can create the cages or givens in a puzzle with the same order of symmetry that you have for the grid layout itself that this generally leads to a more pleasing appearance for the puzzle; but more than this, I find that this tends to follow through with the solving process, and you end up with pieces of the puzzle that feel ’sympathetic’ to one another, since the symmetry leads to related discoveries. However it’s perhaps not clear that this solving benefit carries through to a puzzle this large, and it’s probably the case that a puzzle with entirely random cages would feel just the same to actually solve at this size. But it wouldn’t look as nice!
Coming up in the following days I’m going to experiment in the space between Killer Sudoku and Ken Ken™ – in other words, using more operations than just addition, and possibly allowing repeated numbers in cages (although not on puzzles with 1-9 to place!). I already came across a puzzle called ‘Killer Sudoku Pro’ in the Saturday Telegraph newspaper (UK) – in this they keep the Killer Sudoku rules about not repeating digits in a cage, but specify different operations for cages (in actual fact the rules aren’t stated next to the puzzle in full, but I presume repeated digits are disallowed - it certainly solves okay with that assumption!). I haven’t seen anything precisely like that elsewhere and I thought it was actually quite fun (it wasn’t too hard!) so I’ll definitely try making some of those soon for sure. If you have any other ideas for how to mix these different types together feel free to post a comment!
Good luck!
Consecutive 5-grid Samurai Sudoku
Apr 24th
I thought it would be a nice idea to create a large Consecutive Sudoku for the weekend! And so here one is: a 5-grid Samurai Consecutive Sudoku. As you can see, there are very few givens to start with, so it will hopefully be at least a bit of a challenge! (It shouldn’t be as tricky as the Skyscraper version, at least once you get going!).
I’ve also decided to make Consecutive Sudoku the ‘puzzle of the month’ (”Masterclass”) puzzle in Sudoku Pro issue 45, which should be out in just under 2 months I think. Hopefully I’ll also make a book of them available online soon(ish!).
The rules for this Consecutive Samurai are simple: place 1 to 9 into each row, column and bold-lined 3×3 box of each of the 5 Sudoku grids, whilst also obeying the consecutive constraints – numbers with a white bar between are consecutive, whilst those without a white bar between are not consecutive. ”Consecutive” means that the difference between the values in the two squares is exactly 1: i.e. 1&2, 2&3, 3&4, 4&5, 5&6, 6&7, 7&8 or 8&9.
Good luck!
Shape Sudoku
Apr 23rd
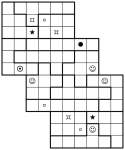
3-grid Shape Jigsaw Sudoku Samurai Stack
I needed to create one of these for a project elsewhere, so I thought I would post a couple of them here too – since I’d gone to the effort to make one at all! It’s not a new Sudoku variation, but just a very simple replacement of the digits 1 to 6 with shapes. None the less, it does make the puzzle notably harder to solve (or maybe that’s just me!). Unless there’s demand I won’t post this variant again, but I thought it would make an interesting change just for once!
I’ve created two examples – one is a simple 6×6 jigsaw, and the other is a 3-grid 6×6 Samurai Stack. In each case place one of each symbol into each 6-square row and column of each underlying 6×6 grid, and also one of each symbol into all of the bold-lined jigsaw shaped pieces.
Good luck!
5-grid Samurai Skyscraper
Apr 20th

Samurai 5-grid Skyscraper puzzle
I thought I’d try one more Skyscraper Samurai Sudoku puzzle – this time a 5-grid variety, or what I think of as the ‘traditional’ Samurai Sudoku format (some people also call this Gattai-5, but I’ve not seen that in print anywhere).
The aim is to place 1 to 9 into each row, column and 3×3 bold-lined box of each of the 5 9×9 Sudoku grids, whilst also obeying the Skyscraper constraints. These tell you the number of digits that can be ’seen’ from the edge of the grid looking in along the adjacent row/column, where higher numbers obscure lower ones. Take a look at a couple of last week’s puzzles if you need more detailed instructions for this constraint.
As has been pointed out in the comments elsewhere, it doesn’t matter whether you consider that the Skyscraper clues apply to the nearest 9×9 grid or to the entire width/height of the row/column they attach to – once the first ‘9′ is reached then there are no higher numbers, and that’s guaranteed to happen within the first 9 squares.
I think this is probably about as large as you want to go with a relatively complex constraint such as Skyscraper, which is why I’ve included quite a few given numbers too – including some which clearly aren’t needed to give the puzzle a unique solution. (But please tell me if I’m wrong about this being big enough – I could always make a much larger one still just to prove that it’s possible!)
This week I plan to try out some other types of consecutive Sudoku variant – there are a couple of moderately-well-known types where you specify certain relationships between adjacent squares, such as ‘x2′ (where one number is twice the adjacent one – a bit like a slightly less-constrained version of consecutive sudoku!). If you have any ideas for other variants, feel free to let me know – I might try them out!
Good luck!
Consecutive Samurai Star
Apr 17th

Samurai Star Consecutive puzzle
If the smaller consecutive puzzles weren’t enough of a challenge then this one should be! There are five overlaid 9×9 grids (including a ‘hidden’ one in the middle) which each need to have 1 to 9 placed into every row, column and bold-lined 3×3 box. On top of this you must obey the consecutive constraints – numbers with a white bar between are consecutive (12, 23, 34, 45, 56, 67, 78 or 89) and those without a bar between are not consecutive.
As you can see, the combination of tightly-overlaid grids and the consecutive marks means that very few givens are needed! Remember that none of these puzzles need ‘complex’ solving logic (you don’t need hidden or naked sets, X-wings or any other even more exotic strategy).
Good luck!
PS If there are any particular Sudoku or Samurai variants you’d like to see, please let me know and I’ll see what I can do!


Recent Comments